Assioma della probabilità

Breves

Thisaxiomcanalsobeexpressedinreverse:"Theeventwiththehighestprobabilityinarandomsamplingisthemostlikelytooccur.)event".

"Onerandomsampling"isatermusedinstatistics.Itallowsyoutorandomlytakeoutoneofmanyobjectswithoutsubjectiveprejudice(insomecases,abatchofsamplingisunifiedasoneExperiment)asasampleforresearch.Thesamplinghereisonlyperformedonce,anditisnotallowedtobedissatisfiedthefirsttime,andthenmakeanothersample.

Verbum "maxime videtur" hasasimplere significationem, andithasataste of "practicam".

Verbum "probabilitas" hasanabstracta ratione "rationalitatis".

Researchhistory

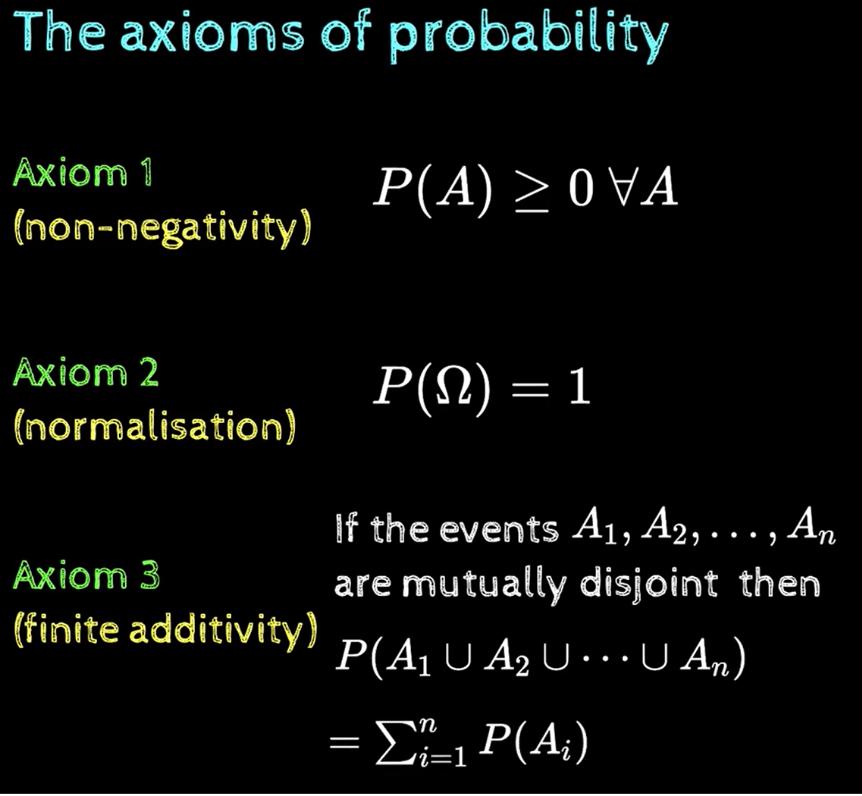
ProbabilityAxioms(ProbabilityAxioms),becauseitsinventorisAndreiKolmogorov,alsoknownasKolmogorovLoveAxiom.WhentheprobabilityP(E)ofaneventEisdefinedinthe"universe"(universe)orthesamplespaceOmegaofallpossiblebasicevents,theprobabilityPmustsatisfythefollowingKolmogorovaxiom.Itcanalsobesaidthatprobabilitycanbeinterpretedasameasuredefinedonthesigmaalgebra($\backslash sigma-Algebra)ofasubsetofthesamplespace.Thosesubsetsareevents,sothatthemeasureofallsetsis$ 1.Thispropertyisimportantbecauseitbringsupthenaturalconceptofconditionalprobability.Foreachnon-zeroprobabilityA,anotherprobabilitycanbedefinedinspace:$ P(B\backslash vertA)=\{P(B\backslash capA)\backslash overP(A)\}Thisisusuallyreadas"givenSettheprobabilityofBwhenA".IftheconditionalprobabilityofBisthesameastheprobabilityofBwhenAisgiven,thenAandBaresaidtobeindependent.Whenthesamplespaceisfiniteorcountableinfinite,theprobabilityfunctioncanalsodefineitsvalueintermsofbasicevents\backslash \{e\_1\backslash \},\backslash \{e\_2\backslash \},...,here\backslash Omega=\backslash \{e\_1,e\_2,...\backslash \}.$$$

Kolmogorov'saxiomsassume quod habebimus abasicset Omega,cuius subset \mathfrak{F}isasigmaalgebra,et AfunctionP quod designat numerum elementorum \ mathfrak{F}.Theementas of mathfrak{F}area subsetof\Omega, quod dicitur "proventus". )\in[0,1].Thatis,theprobabilityofanyeventcanberepresentedbyarealnumberintheintervalfrom0to1.ThesecondaxiomP(\Omega)=1.\,thatis,theprobabilityofacertainbasiceventintheoverallsamplesetis1.Morespecifically,therearenobasiceventsoutsideofthesampleset.Thisisoftenunderestimatedinsomeincorrectprobabilitycalculations;ifyoucannotaccuratelydefinetheentiresampleset,thentheprobabilityofanysubsetcannotbedefined.ThethirdaxiomThecountablesequenceofanypairwisedisjointeventsE_1,E_2,...satisfiesP(E_1\cupE_2\ cup\cdots)=\sumP(E_i).Thatis,theprobabilityofasetofeventsthatisaunionofdisjointsubsetsisthesumoftheprobabilitiesofthosesubsets.Thisisalsocalledσadditivity.Ifthereisoverlapbetweensubsets,thisrelationshipdoesnothold.IfyouwanttounderstandKolmogorov'smethodthroughalgebra,pleaserefertoRandomVariableAlgebra.[Edit]LemmaofProbabilityTheoryFromKolmogorov'saxioms,youcanderivesomeotherusefullawsforcalculatingprobability.P(A\cupB)=P(A)+P( B)-P(A\capB).\,P(\Omega-E)=1-P(E).\,P(A\capB)=P(A)cdotP(B\verA). thisrelationship givesBayes'theorem. Fromthisitcanbeconcluded that AandBareindependentifandonlyifP(A\capB)=P(A)\cdotP(B).\;