Definition
Linear equations whose constant terms are not all zeros are called non-homogeneous linear equations.
The expression of the inhomogeneous linear equations is: Ax=b
Solution
The steps to solve the inhomogeneous linear equations Ax=b:< /p>
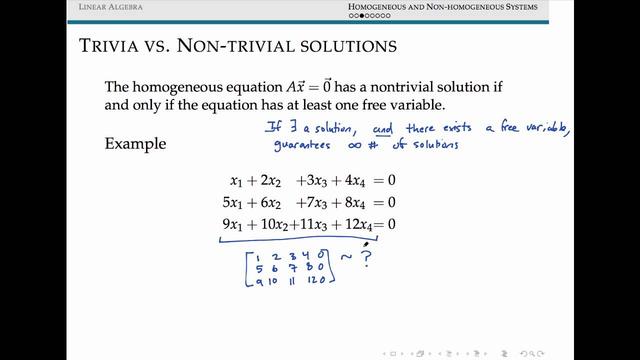
(1) Perform elementary row transformation on augmented matrix B into a row ladder shape. If R(A)
(2) If R(A)=R(B), then B is further reduced to the simplest form of the line.
(3) Let R(A)=R(B)=r; use the remaining nr unknowns (free Unknowns) means, and make the free unknowns equal to
section>, you can write a general solution with nr parameters.Existence of solutions
Inhomogeneous linear equations The necessary and sufficient condition for the solution is: the rank of the coefficient matrix is equal to the rank of the augmented matrix, that is, rank(A)=rank(A, b ) (Otherwise, there is no solution).
The necessary and sufficient condition for inhomogeneous linear equations to have a unique solution is rank(A)=n.
The necessary and sufficient condition for inhomogeneous linear equations to have infinitely many solutions is rank(A)
The structure of the solution
The inhomogeneous linear equations General solution = general solution of homogeneous linear equations + a special solution of non-homogeneous linear equations (η=ζ+η*)
