Axiom pravděpodobnosti

Stručný úvod

Thisaxiomcanalsobeexpressedinreverse:"Theeventwiththehighestprobabilityinarandomsamplingisthemostlikelytooccur.)event".

"Onerandomsampling"isatermusedinstatistics.Itallowsyoutorandomlytakeoutoneofmanyobjectswithoutsubjectiveprejudice(insomecases,abatchofsamplingisunifiedasoneExperiment)asasampleforresearch.Thesamplinghereisonlyperformedonce,anditisnotallowedtobedissatisfiedthefirsttime,andthenmakeanothersample.

Slovo „s největší pravděpodobností se objeví“ má jednoduchý význam a má chuť „praxe“.

Slovo „pravděpodobnost“ má abstraktní význam a chuť „racionality“.

Historie výzkumu

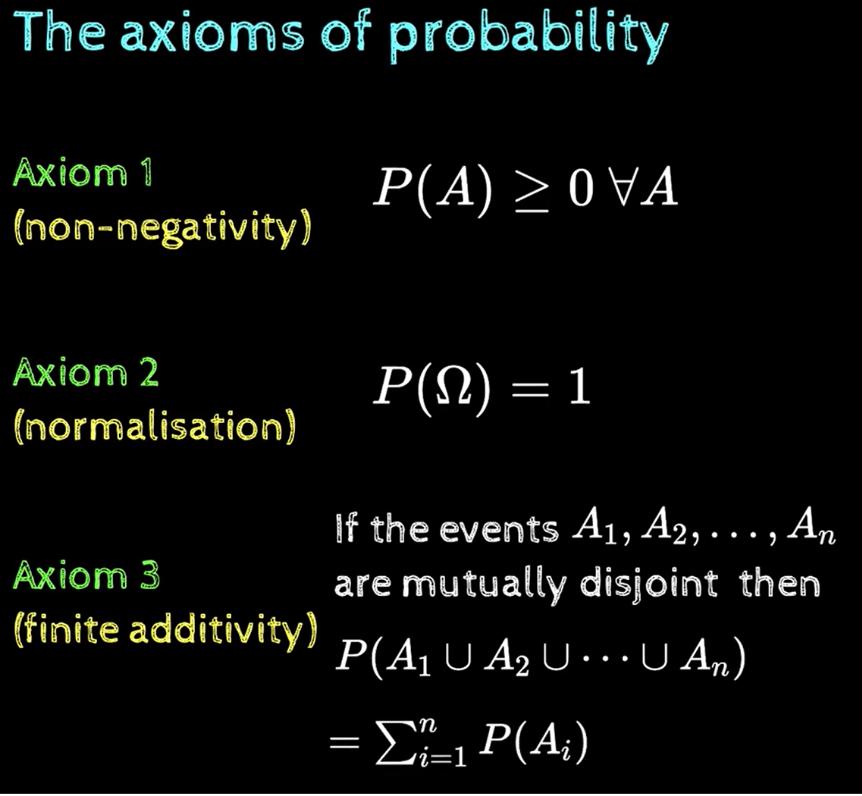
ProbabilityAxioms(ProbabilityAxioms),becauseitsinventorisAndreiKolmogorov,alsoknownasKolmogorovLoveAxiom.WhentheprobabilityP(E)ofaneventEisdefinedinthe"universe"(universe)orthesamplespaceOmegaofallpossiblebasicevents,theprobabilityPmustsatisfythefollowingKolmogorovaxiom.Itcanalsobesaidthatprobabilitycanbeinterpretedasameasuredefinedonthesigmaalgebra($\backslash sigma-Algebra)ofasubsetofthesamplespace.Thosesubsetsareevents,sothatthemeasureofallsetsis$ 1.Thispropertyisimportantbecauseitbringsupthenaturalconceptofconditionalprobability.Foreachnon-zeroprobabilityA,anotherprobabilitycanbedefinedinspace:$ P(B\backslash vertA)=\{P(B\backslash capA)\backslash overP(A)\}Thisisusuallyreadas"givenSettheprobabilityofBwhenA".IftheconditionalprobabilityofBisthesameastheprobabilityofBwhenAisgiven,thenAandBaresaidtobeindependent.Whenthesamplespaceisfiniteorcountableinfinite,theprobabilityfunctioncanalsodefineitsvalueintermsofbasicevents\backslash \{e\_1\backslash \},\backslash \{e\_2\backslash \},...,here\backslash Omega=\backslash \{e\_1,e\_2,...\backslash \}.$$$

Kolmogorovovy saxiomy za předpokladu, že máme základní sadu\Omega, jejíž podmnožina\mathfrak{F}jeasigmaalgebra,afunkceP,kterápřiřazujeskutečnéčísloprvkům\mathfrak{F}.Prvky\mathfrak{F}oblasti,které se nazývá jakákoliv frak\Esta\Esta\E. )\in[0,1].Thatis,theprobabilityofanyeventcanberepresentedbyarealnumberintheintervalfrom0to1.ThesecondaxiomP(\Omega)=1.\,thatis,theprobabilityofacertainbasiceventintheoverallsamplesetis1.Morespecifically,therearenobasiceventsoutsideofthesampleset.Thisisoftenunderestimatedinsomeincorrectprobabilitycalculations;ifyoucannotaccuratelydefinetheentiresampleset,thentheprobabilityofanysubsetcannotbedefined.ThethirdaxiomThecountablesequenceofanypairwisedisjointeventsE_1,E_2,...satisfiesP(E_1\cupE_2\ cup\cdots)=\sumP(E_i).Thatis,theprobabilityofasetofeventsthatisaunionofdisjointsubsetsisthesumoftheprobabilitiesofthosesubsets.Thisisalsocalledσadditivity.Ifthereisoverlapbetweensubsets,thisrelationshipdoesnothold.IfyouwanttounderstandKolmogorov'smethodthroughalgebra,pleaserefertoRandomVariableAlgebra.[Edit]LemmaofProbabilityTheoryFromKolmogorov'saxioms,youcanderivesomeotherusefullawsforcalculatingprobability.P(A\cupB)=P(A)+P( B)-P(A\capB).\,P(\Omega-E)=1-P(E).\,P(A\capB)=P(A)\cdotP(B\vertA).\, tento vztah poskytuje Bayesovu větu. Z toho lze usoudit, že A a Je nezávislá pouze pokud P(A\capB)=P(A)\cdotP(B).\,